magnetic therapy/stimulation and hemoglobin-red blood cell-capillaries; the Magnetic permeability and the magnetic reluctivity and the magnetic susceptibility ; Latex and maxwell's equations; magnetism, magnet, electromagnet, Static and Stationary Magnetic Fields

 In this figure µ (usually µr) is the "dimensionless" number µ/(µ0).
The term of "permeability" was coined in September, 1885 by Oliver Heaviside. 

Rem: 1)all equations are in png with latex in the alt (just right clic on the image to get the code).
2) all the figure contain its URL (auto-bibliography; or sometimes i put the URL below the figure).

This post is a short comment about µ which is the magnetic permeability (and also a compliation of wikipedia and others sites)
It is not easy to define µ and to understand this quantity.
en.Wikipedia : http://en.wikipedia.org/wiki/Magnetic_permeability
and in the case of vacuum: http://en.wikipedia.org/wiki/Vacuum_permeability
Try with other langages.wikipedia  (each x.wikipedia are different encyclopedia and  depand on wikipedians ;)

Permeability is the measure of the ability of a material to support the formation of a magnetic field within itself.

The reciprocal of magnetic permeability is magnetic reluctivity.

Graphical illustration of  the equation B=µH. Simplified comparison of permeabilities for: ferromagnets (μ_f), paramagnets(μ_p), free space(μ₀) and diamagnets (μ_d). This µf is not scaled (in fact it is near of the B-axis).

The auxiliary magnetic field H represents how a magnetic field B influences the organization of magnetic dipoles in a given medium, including dipole migration and magnetic dipole reorientation. Its relation to permeability is

where μ is a scalar if the medium is isotropic or a second rank tensor for an anisotropic medium.
In this post we will assume that µ is a scalar, B and H will be on the same axis (just a comment: in the case of some new special metamaterials we can get a negative µ).

In terms of relative permeability, the magnetic susceptibility is:

χ_m = μ_r − 1.

χ_m, a "dimensionless" quantity, is sometimes called volumetric or bulk susceptibility, to distinguish it from χ_p (magnetic mass or specific susceptibility) and χ_M (molar or molar mass susceptibility).


The magnetization could be modeled as: M = χ_m H


Permeability a constant?
In general, permeability is not a constant (but near of a constant for most of materials), as it can vary with the position in the medium, the frequency of the field applied, humidity, temperature... Permeability as a function of electromagnetic frequency can take on real or complex values.

Table of 'microscopic' equations

Formulation in terms of total charge and current

Name	Differential form	Integral form
Gauss's law
Gauss's law for magnetism
Maxwell–Faraday equation (Faraday's law of induction)
Ampère's circuital law (with Maxwell's correction)

Ref: http://en.wikipedia.org/wiki/Maxwell%27s_equations

µ0 only appears in the Maxwell-Ampère's circuital law. If you use H (not B), it is hidden in H then this equation with B is better ;)

Good luck with units:
permeability is the inductance per unit length. In SI units, permeability is measured in henries per metre (H·m⁻¹ = J/(A²·m) = N A⁻²). H has dimensions current per unit length and is measured in units of amperes per metre (A m⁻¹). The product μH thus has dimensions inductance times current per unit area (H·A/m²). But inductance is magnetic flux per unit current, so the product has dimensions magnetic flux per unit area. This is just the magnetic field B, which is measured in webers (volt-seconds) per square-metre (V·s/m²), or teslas (T).

B the magnetic induction
the Laplace force (a macroscopic force on the wire, when a wire carrying an electrical current is placed in a magnetic field):


tesla=Newton/(A.m)=Newton/(C.(m/s)).
or F=qE then Volt=Newton/(C)
then tesla=Volt/(m/s)

Idl (or J.dV with J Ampere/m2 and dV m3) plays the same role of the electric charge "q" (or densityOfCharge*dV : (C/m3)*m3).

Because the tesla is so large in regards to everyday usage, common engineering practice is to report the strength of magnets in Gauss. 10 G = 1 mT (millitesla).

B perpendicular to F and to dl


H Magnetic field strength
A magnetic dipole is "a closed circulation of electric current" (Maxwell-Ampère's circuital law). The dipole moment has dimensions current times area, units ampere square-metre (A·m2), and magnitude equal to the current around the loop times the area of the loop. H is related to the magnetic dipole density. The H field at a distance from a dipole has magnitude proportional to the dipole moment divided by distance cubed which has dimensions current per unit length.Then without "dimensionless tricks" H is a quantity with many space effects : (A.m2)/m3. H has also a time effect (A= Coulomb/s).

Ampère is a very bad unit to understand something (but it is a very good units in regard to 1Newton and electrotechnics).

-----------------------------------------------
Now we will see the magnitude.
I separate this aspect clearly because it is the most important.
If we dont consider magnets and/or strong stationnary or transient electric currents, all the materials are, in apparence, not "interactive" with magnetic fields.





They are grouped by orders of magnitud:
   0.1 pT  - brain activity, human brain magnetic field:

      1 pT  - cardiac activity:
    20 µT  - strength of magnetic tape near tape head
    31 µT  - strength of Earth's magnetic field at 0° latitude (on the equator)
    58 µT  - strength of Earth's magnetic field at 50° latitude
    0.5 mT  - the suggested exposure limit for cardiac pacemakers
                    by American Conference of Governmental Industrial Hygienists (ACGIH)
    5 mT - the strength of a typical refrigerator magnet
    0.15 T - Sunspots. They are temporary phenomena on the photosphere of the Sun that appear visibly as dark spots compared to surrounding regions. They are caused by intense magnetic activity, which inhibits convection by an effect comparable to the eddy current brake, forming areas of reduced surface temperature.
    1.25 T - Magnetic field intensity at the surface of a neodymium magnet; strength of a modern neodymium-iron-boron (Nd2Fe14B) rare earth magnet. A coin-sized neodymium magnet can lift more than 9 kg, can pinch skin.
    1 T to 2.4 T - coil gap of a typical loudspeaker magnet
    1.5 T to 3 T - strength of medical magnetic resonance imaging systems in practice,
          experimentally up to 17 T
     5 T - The strongest fields encountered from permanent magnets are from Halbach spheres.
  45 T - strongest continuous magnetic field yet produced in a laboratory (Florida State University's National High Magnetic Field Laboratory USA, dec 1999; 34 tons). http://www.magnet.fsu.edu/mediacenter/news/pressreleases/1999december17.html

   91.4 T - strongest (pulsed) magnetic field yet obtained non-destructively in a laboratory (Forschungszentrum Dresden-Rossendorf. http://www.hzdr.de/db/Cms?pOid=33768&pNid=473

   2.8 kT - strongest (pulsed) magnetic field ever obtained (with explosives) in a laboratory (VNIIEF in Sarov, Russia, 1998). DOI: http://dx.doi.org/10.1109/PPC.1999.823621



Ref: http://en.wikipedia.org/wiki/Orders_of_magnitude_%28magnetic_field%29

Magnetic levitation

   16 T - strength used to levitate a frog

              http://www.newscientist.com/article/mg15420771.600-frog-defies-gravity.html
The levitation trick works because giant magnetic fields slightly distort the orbits of electrons in the frog's atoms. The resulting electric current generates a magnetic field in the opposite direction to that of the magnet. A field of 16 teslas created an attractive force strong enough to make the frog float until it made its escape.
The team has also levitated plants, grasshoppers and fish. "If you have a magnet that is big enough, you could levitate a human," says Peter Main, one of the researchers.
He adds that the frog did not seem to suffer any ill effects: "It went back to its fellow frogs looking perfectly happy." 
A live frog levitates inside a 32 mm diameter vertical bore of a Bitter solenoid in a magnetic field of about 16 teslas at the High Field Magnet Laboratory of the Radboud University in Nijmegen the Netherlands:

http://www.youtube.com/watch?v=rotTjRY5lRw

Water possesses diamagnetic properties likewise, although less vivid, which makes the levitation of living beings, containing a large quantity of water, possible. So far the Henri Heim frog levitation experiment within the electromagnetic pair (1997) and the Jung Ming Lu mouse levitation experiment within the electric magnet (2009) have been a success (despite the former assumption of mammals being unable to levitate due to the differing ration of the liquid to the general body mass). 

 http://en.wikipedia.org/wiki/Andre_Geim

"The Frog That Learned to Fly". Radboud University Nijmegen.  For Geim's account of diamagnetic levitation. "Everyone's MagnetismPDF (688 KB). Physics Today. September 1998. pp. 36–39. For the experiment with Berry, see Berry, M. V.; Geim, Andre. (1997). "Of flying frogs and levitrons" PDF (228 KB). European Journal of Physics 18: 307–313.

http://en.wikipedia.org/wiki/Magnetic_levitation
Earnshaw's theorem proves that using only static ferromagnetism it is impossible to stably levitate against gravity, but servomechanisms, the use of diamagnetic materials, superconduction, or systems involving eddy currents permit this to occur.
All materials have diamagnetic properties, but the effect is very weak, and is usually overcome by the object's paramagnetic or ferromagnetic properties, which act in the opposite manner. Any material in which the diamagnetic component is strongest will be repelled by a magnet.
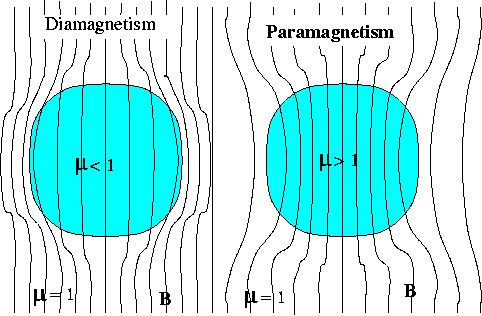
Earnshaw's theorem does not apply to diamagnets. These behave in the opposite manner to normal magnets owing to their relative permeability of μr < 1 (i.e. negative magnetic susceptibility).

Diamagnetic levitation can be used to levitate very light pieces of pyrolytic graphite or bismuth above a moderately strong permanent magnet. As water is predominantly diamagnetic, this technique has been used to levitate water droplets and even live animals, such as a grasshopper, frog and a mouse. However, the magnetic fields required for this are very high, typically in the range of 16 teslas, and therefore create significant problems if ferromagnetic materials are nearby.
The minimum criterion for diamagnetic levitation is

 where:

• ρ is the density of the material
• g is the local gravitational acceleration (−9.8 m/s² on Earth)
• is the rate of change of the magnetic field along the vertical axis.

Assuming ideal conditions along the z-direction of solenoid magnet:

• Water levitates at
• Graphite levitates at

Induced currents

These schemes work due to repulsion due to Lenz's law. When a conductor is presented with a time-varying magnetic field electrical currents in the conductor are set up which create a magnetic field that causes a repulsive effect.

Relative motion between conductors and magnets

If one moves a base made of a very good electrical conductor such as copper, aluminium or silver close to a magnet, an (eddy) current will be induced in the conductor that will oppose the changes in the field and create an opposite field that will repel the magnet (Lenz's law). At a sufficiently high rate of movement, a suspended magnet will levitate on the metal, or vice versa with suspended metal. Litz wire made of wire thinner than the skin depth for the frequencies seen by the metal works much more efficiently than solid conductors.
An especially technologically-interesting case of this comes when one uses a Halbach array instead of a single pole permanent magnet, as this almost doubles the field strength, which in turn almost doubles the strength of the eddy currents. The net effect is to more than triple the lift force. Using two opposed Halbach arrays increases the field even further.
Halbach arrays are also well-suited to magnetic levitation and stabilisation of gyroscopes and electric motor and generator spindles.

Oscillating electromagnetic fields

A conductor can be levitated above an electromagnet (or vice versa) with an alternating current flowing through it. This causes any regular conductor to behave like a diamagnet, due to the eddy currents generated in the conductor. Since the eddy currents create their own fields which oppose the magnetic field, the conductive object is repelled from the electromagnet, and most of the field lines of the magnetic field will no longer penetrate the conductive object.
This effect requires non-ferromagnetic but highly conductive materials like aluminium or copper, as the ferromagnetic ones are also strongly attracted to the electromagnet (although at high frequencies the field can still be expelled) and tend to have a higher resistivity giving lower eddy currents. Again, litz wire gives the best results.
The effect can be used for stunts such as levitating a telephone book by concealing an aluminium plate within it.
At high frequencies (a few tens of kilohertz or so) and kilowatt powers small quantities of metals can be levitated and melted using levitation melting without the risk of the metal being contaminated by the crucible.
-----

Just some comments about magnetic therapy [http://en.wikipedia.org/wiki/Magnet_therapy]:
Magnets produce energy in the form of magnetic fields. Two main types of magnets exist: static or permanent magnets, in which the magnetic field is generated by the spin of electrons within the material itself, and electromagnets, in which a magnetic field is generated when an electric current is applied. Most magnets that are marketed to consumers for health purposes are static magnets of various strengths, typically between 30 and 300 mT. Magnets have been incorporated into arm and leg wraps, mattress pads, necklaces, shoe inserts and bracelets.

The worldwide magnet therapy industry
The worldwide magnet therapy industry totals sales of over a billion dollars per year [http://news.bbc.co.uk/2/hi/health/4582282.stm], including $300 million dollars per year in the United States alone [http://www.csicop.org/si/show/magnet_therapy_a_billion-dollar_boondoggle/].

The ideas of "air du temps":
Even in the magnetic fields used in clinical magnetic resonance imaging, which are many times stronger of 300mT magnet ((i) 0.2 to 9.4 teslas static B and (ii) MegaHertz B), "none" of the claimed effects are observed [http://www.radiologyinfo.org/en/safety/index.cfm?pg=sfty_mr].

The TMS and rTMS is based on transient pulses of 1Teslas/(10-50microseconds) with 5000-8000Ampères/(10-50microseconds) in a coil. In this case some effects are clearly measured on the skin and "sometimes" on the surface of the cortex (e.g. motor cortex). The main effects seem to come from induced electric fields (the Maxwell–Faraday equation expresses that a time variation of B create an electric field: it is the induction).
http://en.wikipedia.org/wiki/Transcranial_magnetic_stimulation

There are many applications of induction (with high levels of B and E) and we need the transduction of eddy currents (courants de Foucault) and of Ohmic losses in special metals ("for induction") to increase the temperature...

There are a lot of controversies about magnet therapy:

Ref:  CMAJ September 25, 2007 vol. 177 no. 7 doi: 10.1503/cmaj.061344 

http://www.cmaj.ca/content/177/7/736.full

Hemoglobin, red blood cell and capillaries
Although hemoglobin, the blood protein that carries oxygen, is weakly  diamagnetic in the oxygenated
and weakly paramagnetic in the deoxygenated state (diamagnetic -> is repulsed by magnetic fields), the magnets used in magnetic therapy seems to be be many orders of magnitude too weak to have any measurable in vivo effect on blood flow.
At 500-600mT (static field), an effect on red blood cells microcirculation (a 40% decrease of RBC velocity  at 600mT @1.5mm in the tissue) was measured: http://www.ncbi.nlm.nih.gov/pubmed/17952798

[Brix, G. et al. Static magnetic fields affect capillary flow of red blood cells in striated skin muscle. Microcirculation 15, 15-26 (2008)]

It has been demonstrated that both normal [10, 11, 14, 35] and sickled [21] human erythrocytes are
aligned by SMF(statif magnetic fields) in cell suspensions. A highly significant orientation was also reported for sickled erythrocytes flowing through a 0.38T field in an in vitro flow apparatus [4]. In a series of experiments [10, 11, 35], Higashi and coworkers found that normal intact RBCs orient with their disk planes parallel to the magnetic field direction. Alignment was detectable at a flux density of 1T and almost 100% of the cells were oriented when exposed to 4T. Since orientation was not influenced by the spin state of hemoglobin (which is diamagnetic in the oxygenated
and paramagnetic in the deoxygenated state), it has been concluded that normal RBCs are oriented primarily due to the anisotropic diamagnetism of cell membrane components. On the other hand, estimations performed for normal RBCs by Schenck [25] indicate that the anisotropic diamagnetic susceptibility of single RBCs is probably too small to orient RBCs flowing in large vessels. These estimations, however, did not take into account that RBCs move in an oriented and deformed state through capillaries, which may change their anisotropic susceptibility (...)
In the case of dynamic RBC clustering, the SMF-induced torque, which increases with the number of anisotropic RBCs coupled, can be much larger than for single RBCs [25]. To obtain a deeper understanding of the observed effect of SMFs on microvascular blood flow, the existing computer models describing dynamic clustering of RBCs in capillaries should be extended to include the physical interaction of the different blood components with an external SMF.
As a first step in this direction, Haik et al. developed a simplified mathematical model, which couples orientation effects of RBCs with the shear stress by introducing a magnetically induced viscosity of blood that adds to the kinetic viscosity. In agreement with their theoretical considerations, the authors experimentally observed an increased viscosity of human blood flowing in a thin plastic tube when exposed to magnetic flux densities between 3 and 10 T as compared to measurements performed in the absence of a SMF [9; Haik Y, Pai V, Chen CJ. (2001). Apparent viscosity of human blood in a high static magnetic field. J Magn Magn Mater 225:180–186.]
Further work will be required to identify potential synergistic or alternative mechanisms by which SMFs are able to affect capillary RBC flow. For example, alterations of the endothelial glycocalyx or of the surface properties of RBCs may play an important role. It is widely recognized that the glycocalyx, a translucent layer with fixed negative charges, has manifold physiological functions. Crucial among these is its role as a hydrodynamic exclusion layer preventing the interaction of proteins in the RBCs and endothelial cell membranes; in modulating leukocyte attachment and rolling; and as a transducer of mechanical forces to the intracellular cytoskeleton in the initiation of intracellular signaling [31] (see also [16,29]). (...)
Muscle capillaries are mainly oriented in parallel and intersected perpendicularly by the magnetic field.(...)
Patients undergoing MR procedures at higher magnetic field strengths occasionally report on mild nausea and headache, which may possibly be related to an altered blood flow pattern [14; Kuchel PW, Coy A, Stilbs P. (1997). NMR “diffusion- diffraction’’ of water revealing alignment of erythrocytes in a magnetic field and their dimensions and membrane transport characteristics. Magn Reson Med 37:637–643].

---[Haik,2001; Apparent viscosity of human blood in a high static magnetic field;   http://dx.doi.org/10.1016/S0304-8853(00)01249-X]
Studying the effect of magnetic field on the blood is of interest to many researchers. Pauling and Coryell [1; 1936] were first to report the diamagnetic susceptibility of oxyhemoglobin and the paramagnetic susceptibility of deoxyhemoglobin. The value for the effective magnetic moments of the Fe²⁺ complex in hemoglobin of red blood cells is derived from their measurements. Higashi et al. [2] studied the orientation of normal erythrocytes in a strong static magnetic field with a maximum field strength of 8 T. The erythrocytes were found to orient with their disc plane parallel to the magnetic field direction. Yamagishi [3] reported a similar behavior of red blood cells at 4 T. Further, Yamagishi [3] found that platelets orient with the applied magnetic field at 3 T. We and others [3] have observed that fibrinogen, one of the plasma proteins, is polymerized and aligns with the applied field already at 4 T. Shalygin and coworkers [4] studied the behavior of erythrocytes in a high-gradient magnetic field. They reported that the susceptibility of the diamagnetic erythrocytes (oxygenated blood in artery) was found to be −(0.13–0.65)×10⁻⁸ cgs emu/cm³ Oe. For the paramagnetic (deoxygenated blood in vein) it was (13–33)×10⁻⁸ cgs emu/cm³ Oe. Similar results were reported by Haik and coworkers [5]. Motta et al. [6] reported orientation of the human hemoglobin when subjected to high magnetic field. Nakano et al. [7] reported that the torque needed to rotate an erythrocyte was very small when the magnetic field was rotating almost parallel to the heme planes in the unit cell, while it was very large when the magnetic field was oriented perpendicular to the heme planes. This demonstrates that the orientation of blood cells when subjected to a magnetic field is due to the magnetic torque. In this orientation, blood cells and the surrounding plasma fluid will interact and, combined with the magnetic force, increase the apparent viscosity of the blood.

--- [Cano,2006;Computer simulation of magnetic properties of human blood; Chemical Physics Letters 432 (2006) 548–552]
Shalygin et al. [6] studied the behaviour of erythrocytes under strong magnetic field gradients. These authors reported a susceptibility for diamagnetic erythrocytes of -(0.13–0.65)x10-8 cgs emu/cm3 Oe and (13–33)x10-8 cgs emu/cm3 Oe for paramagnetic erythrocytes.
From the point of view of the modelling of biological systems it is important to determine which are the basic molecular features that are necessary for a proper modelling. Over the years, primitive models have been very useful in the modelling of complex fluids by computer simulations [9]. In this Letter, we address the description of the magnetic susceptibility of blood using a primitive model comprised of a dipolar hard-spheres fluid (DHS) in the presence of a external field. We study two variations of this model, depending on the physical values used to reproduce the magnetic behaviour of human blood, either red blood cells or reduced hemoglobin molecules.

Following Ref. [8], we are going to consider the susceptibility per ml of substance. The magnetic susceptibility for whole blood, v, is given by
(7)

where χp and χd are the paramagnetic and diamagnetic susceptibility contributions, and mp and md are their fractions, respectively. The paramagnetic contribution arises from the deoxyhemoglobin, whereas the diamagnetic term is basically given by the susceptibility of water molecules, since 60% of blood solution is water [8]. Then, vd ~ 0.6 and χd = 0.6 χwater = -5.4x10-6. The susceptibility of whole human blood is χ = 3.5x10-6 [12]. Using these results in Eq. (7), an estimated value for χp is obtained,
χp =  2:2x10-5  (8)
This value agrees with reported data of χp, that has been determined within the range
-6.07x10-6 ≤ χp ≤ 2.2x10-5 [8,12–17]. Since
χp =nRC χRC  (9)
where nRC is the number of red cells contained within 1 ml of blood, nRC = 5x10^9 [18], and χRC is the magnetic susceptibility of a red blood cell, the magnetic susceptibility of a red blood cell is obtained using Eqs. (8) and (9),
χRC ~ 5x10-15
According to Eq. (5), the magnetization M of a RBC due to the effect of an external magnetic field H is given by
M =χRC H 
Assuming that the magnetization M is basically given by the dipolar moment of the cell, µRC
M = µRC/VRC
where VRC is the volume occupied by a RBC,
VRC = 9.0 x10-11 ml [18], then Eqs. (10)–(12) enable us to have a estimated value of µRC

---------

The relation between life and bio-magnet exists:
Science 23 December 2011: Vol. 334 no. 6063 pp. 1720-1723; DOI: 10.1126/science.1212596
A Cultured Greigite-Producing Magnetotactic Bacterium in a Novel Group of Sulfate-Reducing Bacteria
http://www.sciencemag.org/content/334/6063/1720.abstract
a comment in french: http://www.rtflash.fr/bacterie-produisant-nano-aimants-greigite-enfin-cultivee-en-laboratoire/article


Ref:
http://www.phys.lsu.edu/~jarrell/COURSES/ELECTRODYNAMICS_HTML/course_EM.html
Download: the Latex Source , the full Postscript or PDF notes , Randy's Mathematica examples [1,2,3,4], just the figures, the homework assignment, or the solutions.

Ref: for latex and Equation numbering
http://en.wikibooks.org/wiki/LaTeX/Advanced_Mathematics